An IT2-Based Hybrid Decision-Making Model Using Hesitant Fuzzy Linguistic Term Sets for Selecting the Development Plan of Financial Economics
Corresponding author. Email: hdincer@medipol.edu.tr
- DOI
- 10.2991/ijcis.d.190312.001How to use a DOI?
- Keywords
- Interval type-2 fuzzy DEMATEL; Interval type-2 fuzzy TOPSIS; Hesitant fuzzy linguistic term sets; Economic development plans; Turkey
- Abstract
The novelty of the study is to propose a hybrid IT2 decision-making approach under the hesitant fuzzy linguistic sets for evaluating the criteria and alternatives. For this purpose, the dimensions and criteria are weighted with interval type-2 fuzzy DEMATEL and the economic development plans are ranked by using interval type-2 fuzzy Technique for Order Preference by Similarity to Ideal Solution (TOPSIS) approach. Thus, it is possible to evaluate the multicriteria decision-making problem under the hesitancy more accurately by the extended method.
- Copyright
- © 2019 The Authors. Published by Atlantis Press SARL.
- Open Access
- This is an open access article distributed under the CC BY-NC 4.0 license (http://creativecommons.org/licenses/by-nc/4.0/).
1. INTRODUCTION
Development refers to the improvement of the countries regarding social, cultural, and economic issues [1, 2]. In other words, when there is development in the country, life qualities of the citizens go up. With respect to the economic meaning, development includes low unemployment and high investment and production. On the other side, high-quality education and health system can be given as example for social development. Thus, it is obvious that development of the countries depends on many different aspects [3, 4].
Another important point in this context is that a plan should be defined for development of the country [5]. The main is that there should be a sustainable economic development. Therefore, in order to provide sustainable economic development, many different factors should be taken into the consideration at the same time, such as macroeconomy, health, technology, and legal issues [6, 7]. Hence, if the development policies are implemented without a plan, resources of the country may not be used effectively. This situation can lead to recession in the country [8].
Development plans play a more significant role for emerging economies [9]. Because the main purpose of these countries is to become a developed economy, they try to make high amount of investments. Parallel to this aspect, many actions are also taken by these countries to have better system regarding education, health, and technology. However, in case of taking these actions without a plan, it becomes very difficult to reach sustainable development and this condition may create high amount of losses for these countries [10].
Turkey is also an emerging country which gives importance very much to economic development plans. After the collapse of Ottoman Empire, new Turkish republic was founded in a very difficult environment. There was a recession in the country at these times and labor force was very low due to the wars. In addition to these issues, Turkey has suffered from some different financial crises, such as 1994 and 2001.
Hence, to provide sustainable economic development, Turkey has implemented 10 different 5-years economic development plans. These plans mainly aim to decrease unemployment and inflation rate, increase economic growth and industrial production, and improve social factors [11]. In order to achieve the objectives stated in these plans, many different actions were taken by Turkish governments. It is obvious that some of these plans have better results by comparing with others.
In this study, it is aimed to analyze the performance of these 10 different economic development plans of Turkey. For this purpose, a comparative hybrid approach is proposed to select the best development plans. In this context, 9 different criteria are chosen based on 3 dimensions that are economic, public services, and social factors. Interval type-2 fuzzy DEMATEL approach is considered to weight these dimensions and criteria. Moreover, 10 different economic development plans are ranked with the help of interval type-2 fuzzy Technique for Order Preference by Similarity to Ideal Solution (TOPSIS) approach.
This study has many different novelties. Firstly, a multicriteria decision-making model is firstly used in this study to evaluate 5-years economic development plans. In addition to this issue, it is also the first study in which Turkish economic development plans are ranked according to their performances. Therefore, it can be possible to see whether Turkey is successful to implement development plans over the years. Furthermore, interval type-2 fuzzy DEMATEL methodology is preferred in this study instead of fuzzy analytic hierarchy process (AHP) and fuzzy analytic network process (ANP) methods. The main reason is that DEMATEL methodology provides to make impact and relationship analysis.
There are six different sections in this study. This introduction part contains general information regarding the subject of the study. Also, 10 different 5-years economic development plans of Turkey are explained in the second section. The third section includes literature review. Moreover, the methodologies are identified in the fourth section. In addition, an implementation on Turkey is defined in the fifth section. Finally, conclusions and recommendations are identified in the last section.
2. LITERATURE REVIEW
After the collapse of Ottoman Empire, Turkish Republic was founded in 1923. This new republic faced many difficulties, such as economic and social problems. In these years, some actions were taken by the government like increasing domestic production. Additionally, Turkey implemented 10 different development plans with the aim of providing sustainable economic growth [12].
The first three 5-year development plans cover the years between 1963 and 1977. The First Development Plan focused on unemployment problem and infrastructure investments [13]. Moreover, the Second Development Plan of Turkey aimed to improve industrial sector [14]. In addition to them, the Third Development Plan tried to increase the income level of the country. Also, it was aimed to enhance the production of intermediate goods so that it can be possible to reduce dependence on external resources [15].
Furthermore, the Fourth Development Plan included the improvement of current account balance [16]. However, the Fifth Development Plan of Turkey gave importance to increase the export amount. In order to achieve this objective, it is aimed to minimize government intervention to the market [17]. On the other side, decreasing inflation rate was the main purpose of the Sixth Development Plan [18]. Moreover, the Seventh Development Plan included the collaboration with the world regarding economic activities. Within this framework, European Union integration policies have been given importance [19].
In addition to them, the last three development plans referred to the periods between 2001 and 2018. Because Turkey had a very significant economic crisis in 2001, the Eight Development Plan firstly aimed to decrease budget deficit. Additionally, legal and technological regulations were also the subject of this plan [20]. Moreover, the Ninth Economic Plan of Turkey includes the periods in which there was a hard competition in the world. Also, there was high uncertainty in this period because of the global mortgage crisis occurred in 2008. Therefore, this plan focused on increasing competitive power and providing fair income distribution [21]. Finally, the Tenth Economic Plan of Turkey aimed to increase economic growth, decrease inflation and unemployment rate, and reduce current account deficit problem [22].
Turkey has a purpose to take place among the world’s top 10 economies in 2023. In this context, some actions should be taken to minimize inflation and unemployment rate, increase economic growth and industrial production and improve legal infrastructure. However, it can also be seen that uncertainty increases which causes higher market risks. Hence, by implementing these detail plans, it can be much easier to reach this objective [23].
There are many different studies related to the economic development in the literature. Some of them focused on the relationship between agricultural factors and economic development. Ref. [24] estimated the role of agricultural inputs on economic development. In this scope, 54 different countries are analyzed, and regression methodology is considered in the analysis process. It is concluded that agricultural productivity is a significant indicator of economic development. Similarly, Refs. [25–28] also underlined this situation in their studies.
Additionally, some studies also identified the impact of technological improvement on economic development. Ref. [29] described the factors that have an influence on economic development. In the analysis process, narrative research method is taken into the consideration. They defined that there should be technological improvement in order to reach economic development. Refs. [30–33] determined the significance of technological investment on economic development.
The importance of health policies on economic development was also evaluated by many different researchers. Ref. [34] aimed to analyze the economic development in China. In this study, simultaneous equation model is taken into the consideration to reach the objective. They identified that effective health policies lead to sustainable economic development. Parallel to this study, Refs. [35–40] also determined that for sustainable economic development, health policies should be developed.
In addition to them, the quality of education system in the country also contributed to the economic development according to the many researchers. For example, Ref. [41] defined the leading indicators of economic development. For this purpose, they developed scenarios for the futures. In this study, they concluded that in order to have sustainable economic development, effective health policies should be implemented. Furthermore, Refs. [42–46] are other studies which showed the importance of effective health policies in economic development.
Moreover, some researchers also underlined the importance of development plan to provide sustainability in economic development. For instance, Ref. [47] focused on economic development in the Republic of Korea. In this study, it was underlined that economic development plans have a significant role in the success of this country. Similarly, Refs. [48–54] focused on this topic in their studies and determined that with the help of economic development plan, reaching sustainable economic development becomes much easier.
Furthermore, fuzzy DEMATEL method was used by many different researchers for various subjects. Refs. [55–58] used this approach to evaluate knowledge management. In addition to them, Refs. [59–61] considered DEMATEL method to identify the appropriate strategies during financial crisis periods. This methodology was also considered for the analysis in many different industries, such as energy [62–64], airline [65, 66], and banking [67–69].
Similar to fuzzy DEMATEL approach, fuzzy TOPSIS methodology was also considered in many different studies. As an example, Refs. [70–73] tried to evaluate different energy policies by using this approach. On the other side, Refs. [74–76] examined the effectiveness of many different strategies in the banking sector. Additionally, manufacturing industries were also measured with the help of fuzzy DEMATEL methodology [77–79].
As a result of literature review, it is understood that the subject of development attracted the attentions of many different researchers in the literature. Mainly, the relationship between development and health, technology, and agriculture were evaluated. Additionally, the importance of the economic development plan was also stated in some studies. On the other hand, fuzzy DEMATEL and fuzzy TOPSIS approaches are also very popular in the literature. However, there is not a study which considers the performance of economic development plans by using these methods.
3. METHODOLOGY
3.1. Hesitant Linguistic Term Sets
Subhesitant fuzzy linguistic term set (HFLTS) is defined as a tool of providing the flexibility in linguistic expressions [80]. Decision-makers could prefer to make a decision in several linguistic values to define a membership function [81, 82]. For that, decision-makers under the hesitancy could provide some choices of linguistic scales. This is an extended version of the fuzzy linguistic approach under the hesitancy and it is eased to obtain the data of decision-makers under the hesitancy [83, 84].
Based on the symbolic linguistic model the symbolic linguistic model, S = {S0, S1, …, St} is a linguistic term set and context-free grammar GH is defined as [85]:
A HFLTS is denoted as
3.2. IT2 Fuzzy DEMATEL
DEMATEL represents the expression of “Decision-making trial and evaluation laboratory.” It is a popular type of a multi criteria decision-making model. The main purpose of this approach is to weight different criteria according to their importance [86, 87]. In the literature, AHP and ANP approaches can also make this kind of analysis [88–90]. However, the main difference of DEMATEL method in comparison with these approaches is that impact and relationship analysis can also be performed in this model [91]. DEMATEL methodology can also be considered with interval type-2 fuzzy logic. In the first phase of DEMATEL, decision-makers’ evaluations are converted to the fuzzy sets. Secondly, “the initial direct-relation fuzzy matrix” (Z) is constructed with the help of following equations:
Moreover, the following equations are also used in the process of the normalization of this matrix:
Additionally, “the total influence fuzzy matrix” is calculated in the fourth step with the following equations:
Finally, following equations are considered to construct “the defuzzified total influence matrix”:
3.3. IT2 Fuzzy TOPSIS
The word TOPSIS is obtained from the expression of “Technique for Order Preference by Similarity to Ideal Solution.” This multi criteria decision-making methodology was generated by Hwang and Yoon [92]. With this methodology, it can be possible to rank different alternatives regarding their significance. Both positive (A+) and negative (A−) ideal solutions are calculated in the analysis process of TOPSIS approach. For this purpose, following equations are taken into the consideration:
In the calculation of positive and negative ideal solutions, the weighted values of the defuzzified matrix (vij are used. After that, D+ and D− are calculated as following:
In the final stage, the following equation is considered to identify the closeness coefficient (CCi).
4. AN APPLICATION ON TURKISH DEVELOPMENT PLANS
A hybrid-hesitant decision-making model based on interval type-2 fuzzy sets has been constructed by considering DEMATEL and TOPSIS method, respectively. For this purpose, firstly, The DEMATEL method has been used for weighting the dimensions and criteria of development plan of Turkish financial economics, after that, The TOPSIS has been applied for ranking a set of alternatives defining the development plans between 1963 and 2018. The main reason of selecting interval type-2 fuzzy DEMATEL approach is that this method provides an impact and relationship analysis. Additionally, TOPSIS method also provides accurate solutions by considering uncertainty in order to make decision in complex situation [93–95].
Thus, it is possible to determine the best development plans in Turkey and rank their performances by using the determinants of development plans with the weighted data. Provided data and details of the model construction are summarized in the following sections: Turkey is an emerging country which has a significant geographical location regarding international trade because it is the between the continents of Europe and Asia. Due to this issue, there are lots of different studies in the literature which focuses on Turkey. However, there is not a study that examines this subject with the help of fuzzy logic and multicriteria decision-making methodology.
4.1. Constructing Model
Proposed model starts with defining the problem of multicriteria decision-making model. For this aim, a set of dimensions, criteria, and alternatives have been defined to evaluate with the integrated approach. Table 1 represents the selected dimensions and criteria for the development plans of Turkey.
| Dimensions | Criteria |
|---|---|
| Economic conditions (D1) | Financial system (C1) |
| Fiscal policy (C2) | |
| Investments (C3) | |
| Public services (D2) | Security and justice (C4) |
| Local administrations (C5) | |
| Social and human capacity (D3) | Education (C6) |
| Health (C7) | |
| Social Inclusion (C8) |
Source: Adapted from the development plans of Turkey (1963–2018).
Selected dimensions and criteria for the development plan evaluation.
Table 1 defines three dimensions entitled economic conditions (dimension 1), public services (dimension 2), and social and human capacity (dimension 3) for the development plan evaluation. Additionally, a set of criteria has been represented for each dimension to evaluate the subdimensions of development plan. Financial system (criterion 1), fiscal policy (criterion 2), and investments (criterion 3) are listed as the subdimensions namely, the criteria of economic conditions. In this framework, effective financial system and high foreign and domestic investments contribute to the economic development. Similarly, fair fiscal policies have also positive effect on this situation.
Security and Justice (criterion 4) as well as local administrations (criterion 6) are the subdimensions of public services. In other words, when there is security and justice and local administrations work effectively, it can attract investors and this condition positively affects economic improvement. Finally, education (criterion 6), health (criterion 7), and social inclusion (criterion 8) are defined as a criterion set for the dimension of social and human capacity. Within this context, effective education and health systems in the country increase the living standard of people. Similarly, when people feel that they are the part of the community, it also has a positive influence on the development of the country. Proposed dimensions and criteria have been adapted from 10 development plans of Turkey published in the period of 1963–2018.
However, 10 development plans of Turkey have been selected as a set of alternatives for ranking the best performance among them. Table 2 illustrates the alternatives with their period.
| Alternatives | Period |
|---|---|
| First Development Plan (A1) | (1963–1967) |
| Second Development Plan (A2) | (1968–1972) |
| Third Development Plan (A3) | (1973–1977) |
| Fourth Development Plan (A4) | (1979–1983) |
| Fifth Development Plan (A5) | (1985–1989) |
| Sixth Development Plan (A6) | (1990–1994) |
| Seventh Development Plan (A7) | (1996–2000) |
| Eighth Development Plan (A8) | (2001–2005) |
| Ninth Development Plan (A9) | (2007–2013) |
| Tenth Development Plan (A10) | (2014–2018) |
A set of alternatives for Turkish development plan.
After defining the criteria and alternatives, the decision-maker team has been constructed to provide the linguistic evaluations for the criteria and alternatives. For this purpose, linguistic scales and their fuzzy numbers for the criteria and alternatives are presented in Tables 3 and 4, respectively.
| Linguistic Scales | Interval Type-2 Fuzzy Numbers |
|---|---|
| Very very low (VVL) | ((0,0.1,0.1,0.2;1,1), (0.05,0.1,0.1,0.15;0.9,0.9)) |
| Very low (VL) | ((0.1,0.2,0.2,0.35;1,1), (0.15,0.2,0.2,0.3;0.9,0.9)) |
| Low (L) | ((0.2,0.35,0.35,0.5;1,1), (0.25,0.35,0.35,0.45;0.9,0.9)) |
| Medium (M) | ((0.35,0.5,0.5,0.65;1,1), (0.4,0.5,0.5,0.6;0.9,0.9)) |
| High (H) | ((0.5,0.65,0.65,0.8;1,1), (0.55,0.65,0.65,0.75;0.9,0.9)) |
| Very high (VH) | ((0.65,0.8,0.8,0.9;1,1), (0.7,0.8,0.8,0.85;0.9,0.9)) |
| Very very high (VVH) | ((0.8,0.9,0.9,1;1,1), (0.85,0.9,0.9,0.95;0.9,0.9)) |
Source: Baykasoğlu and Göcük [96].
Linguistic scales and the fuzzy numbers for the criteria.
| Linguistic Scales | Interval Type-2 Fuzzy Numbers |
|---|---|
| Very poor (VP) | ((0,0,0,0.1;1,1), (0,0,0,0.05;0.9,0.9)) |
| Poor (P) | ((0,0.1,0.1,0.3;1,1), (0.05,0.1,0.1,0.2;0.9,0.9)) |
| Medium poor (MP) | ((0.1,0.3,0.3,0.5;1,1), (0.2,0.3,0.3,0.4;0.9,0.9)) |
| Fair (F) | ((0.3,0.5,0.5,0.7;1,1), (0.4,0.5,0.5,0.6;0.9,0.9)) |
| Good (G) | ((0.5,0.7,0.7,0.9;1,1), (0.6,0.7,0.7,0.8;0.9,0.9)) |
| Very good (VG) | ((0.7,0.9,0.9,1;1,1), (0.8,0.9,0.9,0.95;0.9,0.9)) |
| Best (B) | ((0.9,1,1,1;1,1), (0.95,1,1,1;0.9,0.9)) |
Linguistic scales and the fuzzy numbers for the alternatives.
Three decision-makers that are the experts in the field of Turkish financial economics have been appointed to evaluate their linguistic choices and their answers have been considered in the HFLTSs. In this circumstance, three decision-makers evaluated the criterion and alternatives. These experts have at least 10-year experience in this field. The results of the criteria are presented in Table 5.
| Criterion | C1 | C2 | C3 | C4 | C5 | C6 | C7 | C8 |
|---|---|---|---|---|---|---|---|---|
| C1 | - | {M} | {M} | {L, M} | {L, M} | {L, M} | {M, H} | {L, M} |
| C2 | {M, H} | {M} | {L, M} | {M, H} | {M, H} | {M, H} | {M} | |
| C3 | {M} | {M} | - | {M, H} | {M, H} | {M, H} | {M, H} | {L, M} |
| C4 | {L, M} | {L} | {L, M} | - | {L, M} | {L} | {L} | {L, M} |
| C5 | {L} | {L} | {L, M} | {L} | - | {M} | {L, M} | {L, M} |
| C6 | {L} | {L} | {L, M} | {L} | {L, M} | - | {L, M} | {M} |
| C7 | {L, M} | {L} | {L, M} | {L} | {L, M} | {L, M} | - | {L, M} |
| C8 | {L} | {L} | {L, M} | {L, M} | {L, M} | {M, H} | {M} | - |
Hesitant linguistic term sets for the criteria.
The decision-makers have also provided their linguistic evaluations for each alternative with respect to the criteria of the development plan and the hesitant linguistic results for the alternatives are seen in Table 6.
| Criteria/Alternatives | A1 | A2 | A3 | A4 | A5 | A6 | A7 | A8 | A9 | A10 |
|---|---|---|---|---|---|---|---|---|---|---|
| C1 | {MP, F} | {MP, F} | {MP, F} | {F, G} | {F, G} | {F} | {F} | {F, G} | {F, G} | {F, VG} |
| C2 | {MP, F} | {MP, F} | {MP, F} | {F, G} | {F, G} | {F} | {F} | {F, G} | {F, G} | {F, G} |
| C3 | {P, MP} | {P, MP} | {F} | {F} | {F, G} | {F} | {F, G} | {F, G} | {F, G} | {F, VG} |
| C4 | {F, G} | {F, G} | {F} | {F, G} | {F, G} | {F, G} | {F, G} | {F, G} | {F, G} | {F, G} |
| C5 | {F} | {F} | {F} | {F} | {F, G} | {F, G} | {F, G} | {F, G} | {G} | {G} |
| C6 | {MP, F} | {F} | {F} | {F, G} | {F, G} | {F, G} | {F, G} | {F, G} | {F, G} | {F, VG} |
| C7 | {MP, F} | {MP, F} | {F} | {F} | {F, G} | {F, G} | {F, G} | {F, G} | {G, VG} | {G, VG} |
| C8 | {P, MP} | {MP, F} | {F} | {F} | {F, G} | {F, G} | {F, G} | {F, G} | {F, G} | {F, G} |
Hesitant linguistic term sets for the alternatives.
After the defining the criterion and alternative set with their linguistic evaluations, the analysis process continues with calculation of the proposed hybrid model and the details of computation process are given in the following section.
4.2. Results
First stage of the hybrid modelling is to apply IT2-hesitant fuzzy DEMATEL for weighting the criteria and dimensions. In the first step of this stage, the direct-relation matrix has been constructed by using the averaged values of criteria converted into the fuzzy numbers and the results are represented in Table 7.
| C1 | C2 | C3 | C4 | C5 | C6 | C7 | C8 | |
|---|---|---|---|---|---|---|---|---|
| C1 | ((0,0,0,0;1,1), (0,0,0,0;0.90,0.90)) | ((0.35,0.50,0.50,0.65;1,1), (0.40,0.50,0.50,0.60;0.90,0.90)) | ((0.35,0.50,0.50,0.65;1,1), (0.40,0.50,0.50,0.60;0.90,0.90)) | ((0.28,0.43,0.43,0.58;1,1), (0.33,0.43,0.43,0.53;0.90,0.90)) | ((0.28,0.43,0.43,0.58;1,1), (0.33,0.43,0.43,0.53;0.90,0.90)) | ((0.28,0.43,0.43,0.58;1,1), (0.33,0.43,0.43,0.53;0.90,0.90)) | ((0.43,0.58,0.58,0.73;1,1), (0.48,0.58,0.58,0.68;0.90,0.90)) | ((0.28,0.43,0.43,0.58;1,1), (0.33,0.43,0.43,0.53;0.90,0.90)) |
| C2 | ((0.43,0.58,0.58,0.73;1,1), (0.48,0.58,0.58,0.68;0.90,0.90)) | ((0,0,0,0;1,1), (0,0,0,0;0.90,0.90)) | ((0.35,0.50,0.50,0.65;1,1), (0.40,0.50,0.50,0.60;0.90,0.90)) | ((0.28,0.43,0.43,0.58;1,1), (0.33,0.43,0.43,0.53;0.90,0.90)) | ((0.43,0.58,0.58,0.73;1,1), (0.48,0.58,0.58,0.68;0.90,0.90)) | ((0.43,0.58,0.58,0.73;1,1), (0.48,0.58,0.58,0.68;0.90,0.90)) | ((0.43,0.58,0.58,0.73;1,1), (0.48,0.58,0.58,0.68;0.90,0.90)) | ((0.35,0.50,0.50,0.65;1,1), (0.40,0.50,0.50,0.60;0.90,0.90)) |
| C3 | ((0.35,0.50,0.50,0.65;1,1), (0.40,0.50,0.50,0.60;0.90,0.90)) | ((0.35,0.50,0.50,0.65;1,1), (0.40,0.50,0.50,0.60;0.90,0.90)) | ((0,0,0,0;1,1), (0,0,0,0;0.90,0.90)) | ((0.43,0.58,0.58,0.73;1,1), (0.48,0.58,0.58,0.68;0.90,0.90)) | ((0.43,0.58,0.58,0.73;1,1), (0.48,0.58,0.58,0.68;0.90,0.90)) | ((0.43,0.58,0.58,0.73;1,1), (0.48,0.58,0.58,0.68;0.90,0.90)) | ((0.43,0.58,0.58,0.73;1,1), (0.48,0.58,0.58,0.68;0.90,0.90)) | ((0.28,0.43,0.43,0.58;1,1), (0.33,0.43,0.43,0.53;0.90,0.90)) |
| C4 | ((0.28,0.43,0.43,0.58;1,1), (0.33,0.43,0.43,0.53;0.90,0.90)) | ((0.55,0.70,0.70,0.83;1,1), (0.60,0.70,0.70,0.78;0.90,0.90)) | ((0.28,0.43,0.43,0.58;1,1), (0.33,0.43,0.43,0.53;0.90,0.90)) | ((0,0,0,0;1,1), (0,0,0,0;0.90,0.90)) | ((0.28,0.43,0.43,0.58;1,1), (0.33,0.43,0.43,0.53;0.90,0.90)) | ((0.20,0.35,0.35,0.50;1,1), (0.25,0.35,0.35,0.45;0.90,0.90)) | ((0.20,0.35,0.35,0.50;1,1), (0.25,0.35,0.35,0.45;0.90,0.90)) | ((0.28,0.43,0.43,0.58;1,1), (0.33,0.43,0.43,0.53;0.90,0.90)) |
| C5 | ((0.20,0.35,0.35,0.50;1,1), (0.25,0.35,0.35,0.45;0.90,0.90)) | ((0.20,0.35,0.35,0.50;1,1), (0.25,0.35,0.35,0.45;0.90,0.90)) | ((0.28,0.43,0.43,0.58;1,1), (0.33,0.43,0.43,0.53;0.90,0.90)) | ((0.20,0.35,0.35,0.50;1,1), (0.25,0.35,0.35,0.45;0.90,0.90)) | ((0,0,0,0;1,1), (0,0,0,0;0.90,0.90)) | ((0.35,0.50,0.50,0.65;1,1), (0.40,0.50,0.50,0.60;0.90,0.90)) | ((0.28,0.43,0.43,0.58;1,1), (0.33,0.43,0.43,0.53;0.90,0.90)) | ((0.28,0.43,0.43,0.58;1,1), (0.33,0.43,0.43,0.53;0.90,0.90)) |
| C6 | ((0.20,0.35,0.35,0.50;1,1), (0.25,0.35,0.35,0.45;0.90,0.90)) | ((0.20,0.35,0.35,0.50;1,1), (0.25,0.35,0.35,0.45;0.90,0.90)) | ((0.28,0.43,0.43,0.58;1,1), (0.33,0.43,0.43,0.53;0.90,0.90)) | ((0.20,0.35,0.35,0.50;1,1), (0.25,0.35,0.35,0.45;0.90,0.90)) | ((0.28,0.43,0.43,0.58;1,1), (0.33,0.43,0.43,0.53;0.90,0.90)) | ((0,0,0,0;1,1), (0,0,0,0;0.90,0.90)) | ((0.28,0.43,0.43,0.58;1,1), (0.33,0.43,0.43,0.53;0.90,0.90)) | ((0.35,0.50,0.50,0.65;1,1), (0.40,0.50,0.50,0.60;0.90,0.90)) |
| C7 | ((0.28,0.43,0.43,0.58;1,1), (0.33,0.43,0.43,0.53;0.90,0.90)) | ((0.20,0.35,0.35,0.50;1,1), (0.25,0.35,0.35,0.45;0.90,0.90)) | ((0.28,0.43,0.43,0.58;1,1), (0.33,0.43,0.43,0.53;0.90,0.90)) | ((0.20,0.35,0.35,0.50;1,1), (0.25,0.35,0.35,0.45;0.90,0.90)) | ((0.28,0.43,0.43,0.58;1,1), (0.33,0.43,0.43,0.53;0.90,0.90)) | ((0.28,0.43,0.43,0.58;1,1), (0.33,0.43,0.43,0.53;0.90,0.90)) | ((0,0,0,0;1,1), (0,0,0,0;0.90,0.90)) | ((0.28,0.43,0.43,0.58;1,1), (0.33,0.43,0.43,0.53;0.90,0.90)) |
| C8 | ((0.20,0.35,0.35,0.50;1,1), (0.25,0.35,0.35,0.45;0.90,0.90)) | ((0.20,0.35,0.35,0.50;1,1), (0.25,0.35,0.35,0.45;0.90,0.90)) | ((0.28,0.43,0.43,0.58;1,1), (0.33,0.43,0.43,0.53;0.90,0.90)) | ((0.28,0.43,0.43,0.58;1,1), (0.33,0.43,0.43,0.53;0.90,0.90)) | ((0.28,0.43,0.43,0.58;1,1), (0.33,0.43,0.43,0.53;0.90,0.90)) | ((0.43,0.58,0.58,0.73;1,1), (0.48,0.58,0.58,0.68;0.90,0.90)) | ((0.35,0.50,0.50,0.65;1,1), (0.40,0.50,0.50,0.60;0.90,0.90)) | ((0,0,0,0;1,1), (0,0,0,0;0.90,0.90)) |
Direct-relation matrix.
In the second step, the direct-relation matrix has been normalized as seen in Table 8.
| C1 | C2 | C3 | C4 | C5 | C6 | C7 | C8 | |
|---|---|---|---|---|---|---|---|---|
| C1 | ((0,0,0,0;1,1), (0,0,0,0;0.90,0.90)) | ((0.07,0.10,0.10,0.14;1,1), (0.08,0.10,0.10,0.13;0.90,0.90)) | ((0.07,0.10,0.10,0.14;1,1), (0.08,0.10,0.10,0.13;0.90,0.90)) | ((0.06,0.09,0.09,0.12;1,1), (0.07,0.09,0.09,0.11;0.90,0.90)) | ((0.06,0.09,0.09,0.12;1,1), (0.07,0.09,0.09,0.11;0.90,0.90)) | ((0.06,0.09,0.09,0.12;1,1), (0.07,0.09,0.09,0.11;0.90,0.90)) | ((0.09,0.12,0.12,0.15;1,1), (0.10,0.12,0.12,0.14;0.90,0.90)) | ((0.06,0.09,0.09,0.12;1,1), (0.07,0.09,0.09,0.11;0.90,0.90)) |
| C2 | ((0.09,0.12,0.12,0.15;1,1), (0.10,0.12,0.12,0.14;0.90,0.90)) | ((0,0,0,0;1,1), (0,0,0,0;0.90,0.90)) | ((0.07,0.10,0.10,0.14;1,1), (0.08,0.10,0.10,0.13;0.90,0.90)) | ((0.06,0.09,0.09,0.12;1,1), (0.07,0.09,0.09,0.11;0.90,0.90)) | ((0.09,0.12,0.12,0.15;1,1), (0.10,0.12,0.12,0.14;0.90,0.90)) | ((0.09,0.12,0.12,0.15;1,1), (0.10,0.12,0.12,0.14;0.90,0.90)) | ((0.09,0.12,0.12,0.15;1,1), (0.10,0.12,0.12,0.14;0.90,0.90)) | ((0.07,0.10,0.10,0.14;1,1), (0.08,0.10,0.10,0.13;0.90,0.90)) |
| C3 | ((0.07,0.10,0.10,0.14;1,1), (0.08,0.10,0.10,0.13;0.90,0.90)) | ((0.07,0.10,0.10,0.14;1,1), (0.08,0.10,0.10,0.13;0.90,0.90)) | ((0,0,0,0;1,1), (0,0,0,0;0.90,0.90)) | ((0.09,0.12,0.12,0.15;1,1), (0.10,0.12,0.12,0.14;0.90,0.90)) | ((0.09,0.12,0.12,0.15;1,1), (0.10,0.12,0.12,0.14;0.90,0.90)) | ((0.09,0.12,0.12,0.15;1,1), (0.10,0.12,0.12,0.14;0.90,0.90)) | ((0.09,0.12,0.12,0.15;1,1), (0.10,0.12,0.12,0.14;0.90,0.90)) | ((0.06,0.09,0.09,0.12;1,1), (0.07,0.09,0.09,0.11;0.90,0.90)) |
| C4 | ((0.06,0.09,0.09,0.12;1,1), (0.07,0.09,0.09,0.11;0.90,0.90)) | ((0.04,0.07,0.07,0.10;1,1), (0.05,0.07,0.07,0.09;0.90,0.90)) | ((0.06,0.09,0.09,0.12;1,1), (0.07,0.09,0.09,0.11;0.90,0.90)) | ((0,0,0,0;1,1), (0,0,0,0;0.90,0.90)) | ((0.06,0.09,0.09,0.12;1,1), (0.07,0.09,0.09,0.11;0.90,0.90)) | ((0.04,0.07,0.07,0.10;1,1), (0.05,0.07,0.07,0.09;0.90,0.90)) | ((0.04,0.07,0.07,0.10;1,1), (0.05,0.07,0.07,0.09;0.90,0.90)) | ((0.06,0.09,0.09,0.12;1,1), (0.07,0.09,0.09,0.11;0.90,0.90)) |
| C5 | ((0.04,0.07,0.07,0.10;1,1), (0.05,0.07,0.07,0.09;0.90,0.90)) | ((0.04,0.07,0.07,0.10;1,1), (0.05,0.07,0.07,0.09;0.90,0.90)) | ((0.06,0.09,0.09,0.12;1,1), (0.07,0.09,0.09,0.11;0.90,0.90)) | ((0.04,0.07,0.07,0.10;1,1), (0.05,0.07,0.07,0.09;0.90,0.90)) | ((0,0,0,0;1,1), (0,0,0,0;0.90,0.90)) | ((0.07,0.10,0.10,0.14;1,1), (0.08,0.10,0.10,0.13;0.90,0.90)) | ((0.06,0.09,0.09,0.12;1,1), (0.07,0.09,0.09,0.11;0.90,0.90)) | ((0.06,0.09,0.09,0.12;1,1), (0.07,0.09,0.09,0.11;0.90,0.90)) |
| C6 | ((0.04,0.07,0.07,0.10;1,1), (0.05,0.07,0.07,0.09;0.90,0.90)) | ((0.04,0.07,0.07,0.10;1,1), (0.05,0.07,0.07,0.09;0.90,0.90)) | ((0.06,0.09,0.09,0.12;1,1), (0.07,0.09,0.09,0.11;0.90,0.90)) | ((0.04,0.07,0.07,0.10;1,1), (0.05,0.07,0.07,0.09;0.90,0.90)) | ((0.06,0.09,0.09,0.12;1,1), (0.07,0.09,0.09,0.11;0.90,0.90)) | ((0,0,0,0;1,1), (0,0,0,0;0.90,0.90)) | ((0.06,0.09,0.09,0.12;1,1), (0.07,0.09,0.09,0.11;0.90,0.90)) | ((0.07,0.10,0.10,0.14;1,1), (0.08,0.10,0.10,0.13;0.90,0.90)) |
| C7 | ((0.06,0.09,0.09,0.12;1,1), (0.07,0.09,0.09,0.11;0.90,0.90)) | ((0.04,0.07,0.07,0.10;1,1), (0.05,0.07,0.07,0.09;0.90,0.90)) | ((0.06,0.09,0.09,0.12;1,1), (0.07,0.09,0.09,0.11;0.90,0.90)) | ((0.04,0.07,0.07,0.10;1,1), (0.05,0.07,0.07,0.09;0.90,0.90)) | ((0.06,0.09,0.09,0.12;1,1), (0.07,0.09,0.09,0.11;0.90,0.90)) | ((0.06,0.09,0.09,0.12;1,1), (0.07,0.09,0.09,0.11;0.90,0.90)) | ((0,0,0,0;1,1), (0,0,0,0;0.90,0.90)) | ((0.06,0.09,0.09,0.12;1,1), (0.07,0.09,0.09,0.11;0.90,0.90)) |
| C8 | ((0.04,0.07,0.07,0.10;1,1), (0.05,0.07,0.07,0.09;0.90,0.90)) | ((0.04,0.07,0.07,0.10;1,1), (0.05,0.07,0.07,0.09;0.90,0.90)) | ((0.06,0.09,0.09,0.12;1,1), (0.07,0.09,0.09,0.11;0.90,0.90)) | ((0.06,0.09,0.09,0.12;1,1), (0.07,0.09,0.09,0.11;0.90,0.90)) | ((0.06,0.09,0.09,0.12;1,1), (0.07,0.09,0.09,0.11;0.90,0.90)) | ((0.09,0.12,0.12,0.15;1,1), (0.10,0.12,0.12,0.14;0.90,0.90)) | ((0.07,0.10,0.10,0.14;1,1), (0.08,0.10,0.10,0.13;0.90,0.90)) | ((0,0,0,0;1,1), (0,0,0,0;0.90,0.90)) |
Normalized values of the direct-relation matrix.
The following step continues with the computation of the total relation matrix. Table 9 shows the computation results of the total relation matrix.
| C1 | C2 | C3 | C4 | C5 | C6 | C7 | C8 | |
|---|---|---|---|---|---|---|---|---|
| C1 | ((0.04,0.16,0.16,0.76;1,1), (0.07,0.16,0.16,0.41;0.90,0.90)) | ((0.11,0.24,0.24,0.84;1,1), (0.14,0.24,0.24,0.50;0.90,0.90)) | ((0.12,0.26,0.26,0.94;1,1), (0.15,0.26,0.26,0.54;0.90,0.90)) | ((0.10,0.24,0.24,0.85;1,1), (0.13,0.24,0.24,0.50;0.90,0.90)) | ((0.11,0.26,0.26,0.92;1,1), (0.14,0.26,0.26,0.54;0.90,0.90)) | ((0.11,0.26,0.26,0.94;1,1), (0.15,0.26,0.26,0.55;0.90,0.90)) | ((0.14,0.29,0.29,0.97;1,1), (0.17,0.29,0.29,0.58;0.90,0.90)) | ((0.10,0.25,0.25,0.89;1,1), (0.14,0.25,0.25,0.52;0.90,0.90)) |
| C2 | ((0.13,0.29,0.29,0.96;1,1), (0.17,0.29,0.29,0.57;0.90,0.90)) | ((0.05,0.17,0.17,0.79;1,1), (0.07,0.17,0.17,0.42;0.90,0.90)) | ((0.12,0.28,0.28,0.97;1,1), (0.16,0.28,0.28,0.58;0.90,0.90)) | ((0.10,0.26,0.26,0.92;1,1), (0.14,0.26,0.26,0.54;0.90,0.90)) | ((0.14,0.30,0.30,1.01;1,1), (0.18,0.30,0.30,0.61;0.90,0.90)) | ((0.15,0.31,0.31,1.04;1,1), (0.19,0.31,0.31,0.62;0.90,0.90)) | ((0.15,0.31,0.31,1.04;1,1), (0.19,0.31,0.31,0.62;0.90,0.90)) | ((0.12,0.28,0.28,0.98;1,1), (0.16,0.28,0.28,0.58;0.90,0.90)) |
| C3 | ((0.12,0.27,0.27,0.94;1,1), (0.16,0.27,0.27,0.56;0.90,0.90)) | ((0.11,0.26,0.26,0.90;1,1), (0.15,0.26,0.26,0.53;0.90,0.90)) | ((0.06,0.19,0.19,0.85;1,1), (0.08,0.19,0.19,0.46;0.90,0.90)) | ((0.13,0.28,0.28,0.94;1,1), (0.17,0.28,0.28,0.56;0.90,0.90)) | ((0.14,0.30,0.30,1.01;1,1), (0.18,0.30,0.30,0.60;0.90,0.90)) | ((0.15,0.31,0.31,1.04;1,1), (0.19,0.31,0.31,0.62;0.90,0.90)) | ((0.15,0.31,0.31,1.04;1,1), (0.18,0.31,0.31,0.62;0.90,0.90)) | ((0.11,0.27,0.27,0.96;1,1), (0.15,0.27,0.27,0.56;0.90,0.90)) |
| C4 | ((0.09,0.22,0.22,0.78;1,1), (0.12,0.22,0.22,0.46;0.90,0.90)) | ((0.07,0.20,0.20,0.74;1,1), (0.10,0.20,0.20,0.43;0.90,0.90)) | ((0.09,0.22,0.22,0.81;1,1), (0.12,0.22,0.22,0.47;0.90,0.90)) | ((0.03,0.13,0.13,0.66;1,1), (0.05,0.13,0.13,0.35;0.90,0.90)) | ((0.09,0.23,0.23,0.83;1,1), (0.13,0.23,0.23,0.49;0.90,0.90)) | ((0.08,0.22,0.22,0.84;1,1), (0.12,0.22,0.22,0.49;0.90,0.90)) | ((0.08,0.22,0.22,0.84;1,1), (0.12,0.22,0.22,0.48;0.90,0.90)) | ((0.09,0.22,0.22,0.81;1,1), (0.12,0.22,0.22,0.47;0.90,0.90)) |
| C5 | ((0.08,0.21,0.21,0.78;1,1), (0.11,0.21,0.21,0.45;0.90,0.90)) | ((0.07,0.20,0.20,0.74;1,1), (0.10,0.20,0.20,0.43;0.90,0.90)) | ((0.09,0.23,0.23,0.82;1,1), (0.12,0.23,0.23,0.48;0.90,0.90)) | ((0.07,0.20,0.20,0.77;1,1), (0.11,0.20,0.20,0.44;0.90,0.90)) | ((0.04,0.15,0.15,0.73;1,1), (0.06,0.15,0.15,0.39;0.90,0.90)) | ((0.11,0.25,0.25,0.84;1,1), (0.15,0.25,0.25,0.52;0.90,0.90)) | ((0.10,0.24,0.24,0.86;1,1), (0.13,0.24,0.24,0.50;0.90,0.90)) | ((0.09,0.23,0.23,0.82;1,1), (0.12,0.23,0.23,0.49;0.90,0.90)) |
| C6 | ((0.08,0.21,0.21,0.78;1,1), (0.11,0.21,0.21,0.45;0.90,0.90)) | ((0.07,0.20,0.20,0.75;1,1), (0.10,0.20,0.20,0.43;0.90,0.90)) | ((0.09,0.23,0.23,0.82;1,1), (0.12,0.23,0.23,0.48;0.90,0.90)) | ((0.07,0.20,0.20,0.77;1,1), (0.11,0.20,0.20,0.44;0.90,0.90)) | ((0.10,0.23,0.23,0.84;1,1), (0.13,0.23,0.23,0.49;0.90,0.90)) | ((0.04,0.16,0.16,0.76;1,1), (0.07,0.16,0.16,0.41;0.90,0.90)) | ((0.10,0.24,0.24,0.86;1,1), (0.13,0.24,0.24,0.50;0.90,0.90)) | ((0.11,0.24,0.24,0.83;1,1), (0.14,0.24,0.24,0.49;0.90,0.90)) |
| C7 | ((0.09,0.22,0.22,0.78;1,1), (0.12,0.22,0.22,0.46;0.90,0.90)) | ((0.07,0.20,0.20,0.75;1,1), (0.10,0.20,0.20,0.43;0.90,0.90)) | ((0.09,0.23,0.23,0.82;1,1), (0.12,0.23,0.23,0.48;0.90,0.90)) | ((0.07,0.20,0.20,0.77;1,1), (0.11,0.20,0.20,0.45;0.90,0.90)) | ((0.10,0.23,0.23,0.84;1,1), (0.13,0.23,0.23,0.49;0.90,0.90)) | ((0.10,0.24,0.24,0.86;1,1), (0.13,0.24,0.24,0.51;0.90,0.90)) | ((0.04,0.16,0.16,0.76;1,1), (0.07,0.16,0.16,0.41;0.90,0.90)) | ((0.09,0.23,0.23,0.82;1,1), (0.12,0.23,0.23,0.48;0.90,0.90)) |
| C8 | ((0.08,0.21,0.21,0.78;1,1), (0.11,0.21,0.21,0.45;0.90,0.90)) | ((0.07,0.21,0.21,0.78;1,1), (0.11,0.21,0.21,0.45;0.90,0.90)) | ((0.10,0.24,0.24,0.85;1,1), (0.13,0.24,0.24,0.50;0.90,0.90)) | ((0.09,0.23,0.23,0.81;1,1), (0.12,0.23,0.23,0.48;0.90,0.90)) | ((0.10,0.24,0.24,0.87;1,1), (0.13,0.24,0.24,0.51;0.90,0.90)) | ((0.13,0.28,0.28,0.92;1,1), (0.17,0.28,0.28,0.55;0.90,0.90)) | ((0.12,0.26,0.26,0.97;1,1), (0.15,0.26,0.26,0.54;0.90,0.90)) | ((0.04,0.16,0.16,0.74;1,1), (0.07,0.16,0.16,0.40;0.90,0.90)) |
Total-relation matrix.
At the final step of IT2-hesitant fuzzy DEMATEL, the defuzzification process has been applied for providing the impact and relation degrees of each criterion and the final weighting results of them. The defuzzified values are represented in Table 10.
| C1 | C2 | C3 | C4 | C5 | C6 | C7 | C8 | r | y | r+ y | r− y | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| C1 | 0.24 | 0.31 | 0.34 | 0.31 | 0.34 | 0.34 | 0.37 | 0.33 | 2.58 | 2.36 | 4.94 | 0.21 |
| C2 | 0.37 | 0.25 | 0.36 | 0.34 | 0.39 | 0.40 | 0.40 | 0.36 | 2.86 | 2.22 | 5.08 | 0.64 |
| C3 | 0.35 | 0.34 | 0.27 | 0.36 | 0.39 | 0.40 | 0.40 | 0.35 | 2.85 | 2.47 | 5.32 | 0.38 |
| C4 | 0.28 | 0.26 | 0.29 | 0.20 | 0.30 | 0.30 | 0.30 | 0.29 | 2.23 | 2.32 | 4.55 | −0.09 |
| C5 | 0.27 | 0.26 | 0.30 | 0.27 | 0.23 | 0.33 | 0.31 | 0.30 | 2.27 | 2.56 | 4.83 | −0.30 |
| C6 | 0.27 | 0.26 | 0.30 | 0.27 | 0.31 | 0.24 | 0.31 | 0.31 | 2.27 | 2.67 | 4.94 | −0.40 |
| C7 | 0.29 | 0.26 | 0.30 | 0.27 | 0.31 | 0.31 | 0.23 | 0.30 | 2.27 | 2.66 | 4.94 | −0.39 |
| C8 | 0.29 | 0.27 | 0.31 | 0.30 | 0.32 | 0.35 | 0.34 | 0.23 | 2.41 | 2.47 | 4.88 | −0.06 |
Defuzzified values and impact-relation results of criteria.
According to the results of (r+ y), C3 is the most important factor as C4 has the weakest importance in the criterion set. However, the values of (y − x) demonstrate that C2 is the most influencing factor in the criteria set whereas C6 is the most influenced criteria of the development plan. Moreover, the impact-relation map among the criterion set of the development plan is illustrated. For that, the threshold item that is the averaged value of the defuzzified matrix is appointed to determine the impact directions among the criteria and the results are represented in Figure 1.
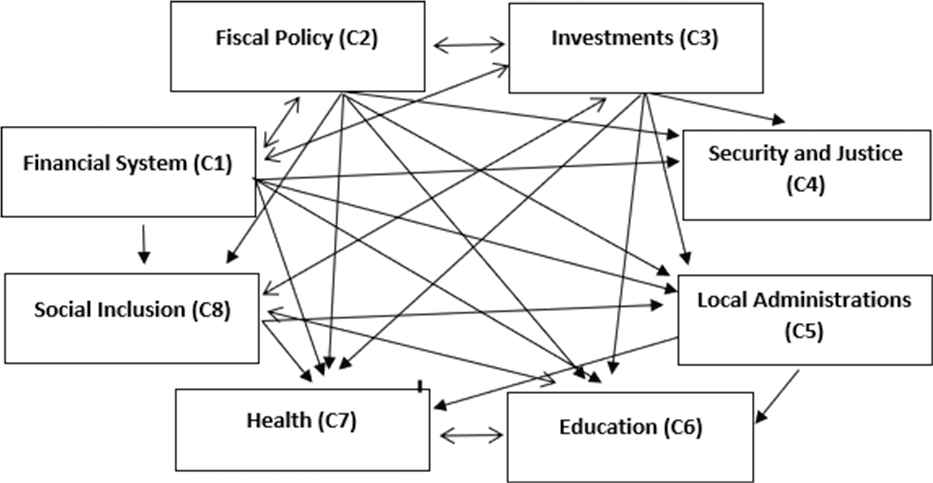
Impact and relation map of the criteria.
As seen in Figure 1, C1, C2, and C3 have completely impact on the criterion set. However, C4 has no impact on the other criteria. Some of criteria has a mutual relation between each other such as C1 and C2, C2 and C3, C1 and C3. Additionally, Table 11 defines the local and global weights of development plan factors.
| Local Dimensions Weights | Criteria | Local Weights | Global Weights |
|---|---|---|---|
| (D1) 0.389 | C1 | 0.322 | 0.125 |
| C2 | 0.331 | 0.129 | |
| C3 | 0.347 | 0.135 | |
| (D2) 0.238 | C4 | 0.485 | 0.115 |
| C5 | 0.515 | 0.122 | |
| (D3) 0.374 | C6 | 0.335 | 0.125 |
| C7 | 0.335 | 0.125 | |
| C8 | 0.331 | 0.124 |
Local and global weights of factors.
Table 11 shows that dimension 1 is the most important dimension as dimension 2 has relatively weakest importance in the dimension set. The second stage of the hybrid analysis continues with IT2-hesitant fuzzy TOPSIS for ranking alternatives. Firstly, the decision matrix has been converted into the averaged fuzzy numbers to obtain the fuzzy decision matrix under the hesitancy. Table 12 represents the fuzzy decision matrix.
| A1 | A2 | A3 | A4 | A5 | A6 | A7 | A8 | A9 | A10 | |
|---|---|---|---|---|---|---|---|---|---|---|
| C1 | ((0.20,0.40,0.60,0.97;1,1), 0.30,0.40,0.40,0.50;0.90,0.90) | ((0.20,0.75,0.75,0.45;1,1), (0.30,0.40,0.40,0.50;0.90,0.90)) | ((0.20,0.40,0.40,0.60;1,1), 0.30,0.40,0.40,0.50;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.30,0.50,0.50,0.70;1.00,1.00), (0.40,0.50,0.50,0.60;0.90,0.90)) | ((0.30,0.50,0.50,0.70;1.00,1.00), (0.40,0.50,0.50,0.60;0.90,0.90)) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.50,0.70,0.70,0.85;1,1), (0.60,0.70,0.70,0.80;0.90,0.90)) |
| C2 | ((0.20,0.40,0.60,0.97;1,1), 0.30,0.40,0.40,0.50;0.90,0.90) | ((0.20,0.75,0.75,0.45;1,1), (0.30,0.40,0.40,0.50;0.90,0.90)) | ((0.20,0.40,0.40,0.60;1,1), 0.30,0.40,0.40,0.50;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.30,0.50,0.50,0.70;1.00,1.00), (0.40,0.50,0.50,0.60;0.90,0.90)) | ((0.30,0.50,0.50,0.70;1.00,1.00), (0.40,0.50,0.50,0.60;0.90,0.90)) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) |
| C3 | ((0.05,0.20,0.20,0.40;1,1), (0.13,0.20,0.20,0.30;0.90,0.90) | ((0.05,0.65,0.65,0.28;1,1), (0.13,0.20,0.20,0.30;0.90,0.90) | ((0.30,0.50,0.50,0.70;1.00,1.00), (0.40,0.50,0.50,0.60;0.90,0.90)) | ((0.30,0.50,0.50,0.70;1.00,1.00), (0.40,0.50,0.50,0.60;0.90,0.90)) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.30,0.50,0.50,0.70;1.00,1.00), (0.40,0.50,0.50,0.60;0.90,0.90)) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.50,0.70,0.70,0.85;1,1), (0.60,0.70,0.70,0.80;0.90,0.90)) |
| C4 | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.75,0.75,0.65;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.30,0.50,0.50,0.70;1.00,1.00), (0.40,0.50,0.50,0.60;0.90,0.90)) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) |
| C5 | ((0.30,0.50,0.50,0.70;1.00,1.00), (0.40,0.50,0.50,0.60;0.90,0.90)) | ((0.30,0.75,0.75,0.70;1.00,1.00), (0.40,0.50,0.50,0.60;0.90,0.90)) | ((0.30,0.50,0.50,0.70;1.00,1.00), (0.40,0.50,0.50,0.60;0.90,0.90)) | ((0.30,0.50,0.50,0.70;1.00,1.00), (0.40,0.50,0.50,0.60;0.90,0.90)) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.50,0.70,0.70,0.90;1,1), (0.60,0.70,0.70,0.80;0.90,0.90)) | ((0.50,0.70,0.70,0.90;1,1), (0.60,0.70,0.70,0.80;0.90,0.90)) |
| C6 | ((0.20,0.40,0.60,0.97;1,1), 0.30,0.40,0.40,0.50;0.90,0.90) | ((0.30,0.75,0.75,0.55;1.00,1.00), (0.40,0.50,0.50,0.60;0.90,0.90)) | ((0.30,0.50,0.50,0.70;1.00,1.00), (0.40,0.50,0.50,0.60;0.90,0.90)) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.50,0.70,0.70,0.85;1,1), (0.60,0.70,0.70,0.80;0.90,0.90)) |
| C7 | ((0.20,0.40,0.60,0.97;1,1), 0.30,0.40,0.40,0.50;0.90,0.90) | ((0.20,0.65,0.65,0.45;1,1), (0.30,0.40,0.40,0.50;0.90,0.90)) | ((0.30,0.50,0.50,0.70;1.00,1.00), (0.40,0.50,0.50,0.60;0.90,0.90)) | ((0.30,0.50,0.50,0.70;1.00,1.00), (0.40,0.50,0.50,0.60;0.90,0.90)) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.60,0.80,0.80,0.95;1,1), (0.70,0.80,0.80,0.88;0.90,0.90)) | ((0.60,0.80,0.80,0.95;1,1), (0.70,0.80,0.80,0.88;0.90,0.90)) |
| C8 | ((0.05,0.20,0.20,0.40;1,1), (0.13,0.20,0.20,0.30;0.90,0.90) | ((0.20,0.75,0.75,0.45;1,1), (0.30,0.40,0.40,0.50;0.90,0.90)) | ((0.30,0.50,0.50,0.70;1.00,1.00), (0.40,0.50,0.50,0.60;0.90,0.90)) | ((0.30,0.50,0.50,0.70;1.00,1.00), (0.40,0.50,0.50,0.60;0.90,0.90)) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) | ((0.40,0.60,0.60,0.80;1,1), (0.50,0.60,0.60,0.70;0.90,0.90) |
Fuzzy decision matrix.
The following process is to get the defuzzified values of the decision matrix. Provided values are shown in Table 13.
| A1 | A2 | A3 | A4 | A5 | A6 | A7 | A8 | A9 | A10 | |
|---|---|---|---|---|---|---|---|---|---|---|
| C1 | 6.07 | 6.62 | 6.07 | 7.27 | 7.27 | 6.67 | 6.67 | 7.27 | 7.27 | 7.85 |
| C2 | 6.07 | 6.62 | 6.07 | 7.27 | 7.27 | 6.67 | 6.67 | 7.27 | 7.27 | 7.27 |
| C3 | 4.93 | 5.65 | 6.67 | 6.67 | 7.27 | 6.67 | 7.27 | 7.27 | 7.27 | 7.85 |
| C4 | 7.27 | 7.49 | 6.67 | 7.27 | 7.27 | 7.27 | 7.27 | 7.27 | 7.27 | 7.27 |
| C5 | 6.67 | 7.15 | 6.67 | 6.67 | 7.27 | 7.27 | 7.27 | 7.27 | 7.87 | 7.87 |
| C6 | 6.07 | 7.05 | 6.67 | 7.27 | 7.27 | 7.27 | 7.27 | 7.27 | 7.27 | 7.85 |
| C7 | 6.07 | 6.45 | 6.67 | 6.67 | 7.27 | 7.27 | 7.27 | 7.27 | 8.45 | 8.45 |
| C8 | 4.93 | 6.62 | 6.67 | 6.67 | 7.27 | 7.27 | 7.27 | 7.27 | 7.27 | 7.27 |
Defuzzified values of the decision matrix.
Weights of the criteria from IT2-hesitant fuzzy DEMATEL have been used for the weighted defuzzified decision matrix and the results are presented in Table 14.
| A1 | A2 | A3 | A4 | A5 | A6 | A7 | A8 | A9 | A10 | |
|---|---|---|---|---|---|---|---|---|---|---|
| C1 | 0.76 | 0.83 | 0.76 | 0.91 | 0.91 | 0.84 | 0.84 | 0.91 | 0.91 | 0.98 |
| C2 | 0.78 | 0.85 | 0.78 | 0.94 | 0.94 | 0.86 | 0.86 | 0.94 | 0.94 | 0.94 |
| C3 | 0.66 | 0.76 | 0.90 | 0.90 | 0.98 | 0.90 | 0.98 | 0.98 | 0.98 | 1.06 |
| C4 | 0.84 | 0.86 | 0.77 | 0.84 | 0.84 | 0.84 | 0.84 | 0.84 | 0.84 | 0.84 |
| C5 | 0.82 | 0.88 | 0.82 | 0.82 | 0.89 | 0.89 | 0.89 | 0.89 | 0.96 | 0.96 |
| C6 | 0.76 | 0.88 | 0.83 | 0.91 | 0.91 | 0.91 | 0.91 | 0.91 | 0.91 | 0.98 |
| C7 | 0.76 | 0.81 | 0.83 | 0.83 | 0.91 | 0.91 | 0.91 | 0.91 | 1.06 | 1.06 |
| C8 | 0.61 | 0.82 | 0.82 | 0.82 | 0.90 | 0.90 | 0.90 | 0.90 | 0.90 | 0.90 |
Weighted decision matrix.
At the final step of the second stage, the values for the positive and negative ideal solution as well as the relative closeness have been computed. Table 15 shows the values and ranking results for the alternatives of development plan.
| Alternatives | Di+ | Di− | RCi | Ranking |
|---|---|---|---|---|
| A1 | 0.688 | 0.069 | 0.091 | 10 |
| A2 | 0.453 | 0.305 | 0.402 | 9 |
| A3 | 0.454 | 0.337 | 0.426 | 8 |
| A4 | 0.336 | 0.426 | 0.559 | 7 |
| A5 | 0.210 | 0.535 | 0.718 | 3 |
| A6 | 0.293 | 0.454 | 0.608 | 6 |
| A7 | 0.258 | 0.501 | 0.660 | 5 |
| A8 | 0.210 | 0.535 | 0.718 | 3 |
| A9 | 0.131 | 0.607 | 0.822 | 2 |
| A10 | 0.025 | 0.691 | 0.965 | 1 |
RCi, relative closeness index.
Ranking results for alternatives.
Table 15 demonstrates that A10 is the best development plan between 1963 and 2018 while A1 has the last rank in the alternative set. However, last three development plan in Turkey have the best seats during this period. The results are coherent for the historical development of Turkey except the fifth Development Plan between 1985 and 1989 (A5).
5. DISCUSSIONS AND CONCLUSIONS
Having economic development plan is important especially for emerging economies. Since they aim to be a developed country, they try make many different actions to reach this purpose quickly. For example, Turkey is an emerging economy which implemented 10 different 5-year economic development plans for the years between 1963 and 2018. They mainly aim to minimize unemployment and inflation rate, increase technological investment, economic growth, and industrial production and improve legal, health, and education systems.
This study aims to evaluate the performance of these 10 different economic development plans of Turkey. In this framework, three dimensions and nine criteria are identified. They are weighted with the help of interval type-2 fuzzy DEMATEL approach. The results show that economic conditions play the most significant role whereas the dimension of public services is on the last rank. This explains that so as to reach sustainable development countries firstly focus on economic conditions. The importance of the economic issues for success of the development plan in emerging economies were also emphasized in many different studies in the literature [98–100].
Similarly, as a result of interval type-2 DEMATEL analysis, the criteria of investment, fiscal policy, and financial systems have the highest weights. It is defined that countries should take some actions to attract the investors. In this circumstance, there should be tax advantage for both domestic and foreign investors. High investments make contribution to increase economic growth and decrease unemployment rate. This condition positively affects the development of the countries.
Another important point is that fair fiscal policies should be implemented. With the help of these fair fiscal policies, it can be much easier to increase savings and attract the investors. Finally, effective financial system also contributes to effectiveness of the fund transferring from fund suppliers to the fund demanders. It has also positive influence on economic development of the country. Similarly, Refs. [101–103] also underlined that when financial system becomes more effective, it can be possible to reach sustainable economic growth.
In addition to these issues, 10 different 5-year economic development plans of Turkey are ranked by using interval type-2 fuzzy TOPSIS approach. In this context, the weights of the dimensions and criteria calculated by using interval type-2 fuzzy DEMATEL are also considered. The findings explain that last three development plans (2001–2018) in Turkey have higher performance in comparison with the previous ones.
These plans were implemented in Turkey successfully after suffering from the financial crisis in 2001 and during the global crisis of 2008. In these plans, it was aimed to decrease unemployment and inflation rates. Additionally, more effective health and infrastructure systems were implemented. According to the results of this study, it is understood that these plans contributed the development of Turkey more by comparing with the earlier development plans.
This study aimed to focus on a very important topic in financial economies. Additionally, it is believed that using interval type-2 fuzzy DEMATEL and TOPSIS methods firstly increases the originality of this study. Nevertheless, in the future studies, many different emerging economies can be taken into the consideration by using different approaches, such as interval type-2 fuzzy VIKOR and interval type-2 fuzzy QUALIFLEX.
REFERENCES
Cite this article
TY - JOUR AU - Hasan Dincer AU - Secil Senel Uzunkaya AU - Serhat Yüksel PY - 2019 DA - 2019/03/06 TI - An IT2-Based Hybrid Decision-Making Model Using Hesitant Fuzzy Linguistic Term Sets for Selecting the Development Plan of Financial Economics JO - International Journal of Computational Intelligence Systems SP - 460 EP - 473 VL - 12 IS - 2 SN - 1875-6883 UR - https://doi.org/10.2991/ijcis.d.190312.001 DO - 10.2991/ijcis.d.190312.001 ID - Dincer2019 ER -
